Teleportation of electricity

Up: Topics

Teleportation of electricity

This article summarizes some recent work from our group. For another perspective on the same research, see PhysRev Focus (6 February 2004).
Carlo Beenakker

Teleportation is the transfer of a quantum mechanical state between two particles that can only communicate by classical means. Because the transfer takes place without exchange of matter, it is reminiscent of the well known Beam me up! from the StarTrek television series. Teleportation of isolated particles was invented ten years ago [1] and demonstrated for photons in free space [2]. We have found a way to teleport electrical charge in the solid state [3]. This discovery could be used to transfer quantum mechanical bits (qubits) in a quantum computer.
Electron meets hole

There exist two types of charge carriers in the solid state, electrons and holes. Because they are oppositely charged, they can only exist simultaneously if they are separated from each other by an insulating barrier. If the barrier still passes a small current and an electron meets a hole, then both are annihilated. We have discovered that this need not be the end of the story. Under special circumstances the electron can continue its existence at a distant location by teleportation.
The meeting of an electron and a hole is illustrated is figure 1. Both particles live in the conduction band of a metal or semiconductor. At low temperature all energy levels are filled with electrons up to a maximal energy. This "sea" of electrons is called the Fermi sea and the maximal energy level is the Fermi level. The fully filled Fermi sea is in equilibrium and therefore carries no electrical current. To pass a current you need excitations. These are filled states (electrons) above the Fermi level or empty states (holes) below it. The meeting takes place at an insulating barrier, which plays the role of a sluice: The Fermi level is a little higher on one side of the barrier than on the other, so that the hole is elevated to the same energy as the electron.

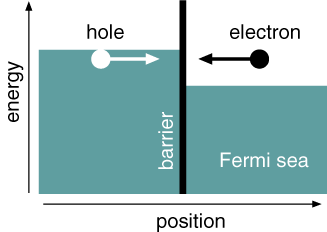
Figure 1: An electron meets a hole.

Usually the electron and the hole are reflected by the barrier, but each time they meet there is a small probability that the electron will tunnel through the barrier and fall into the hole at the other side. Then both particles disappear without leaving a trace. End of story. Unless ... the hole had been entangled in the past with another electron, at some distant location in the material. I will refer to this second, distant electron as the "heavenly" electron and to the first electron as the "earthly" electron.
Entangled electron-hole pair

Entanglement is a quantum mechanical correlation between the spins. The spin of the hole is, on the one hand, oriented completely isotropically and, on the other hand, fully correlated with the spin of the heavenly electron. In classical mechanics this would be impossible, but not so in quantum mechanics. The wave function that describes such an entangled state of hole and electron is $(\vert\!\uparrow\uparrow\rangle+\vert\!\downarrow\downarrow\rangle)/\sqrt{2}$ . It is a superposition of two states; In the first state electron and hole have both spin $\uparrow$ and in the second state they have both spin $\downarrow$ . Such an entangled state is created naturally when the heavenly electron tunnels through a barrier and leaves behind a hole with the same spin [4]. See figure 2.

Figure 2: Creation of an entangled electron-hole pair. Both spins are isotropically distributed but perfectly correlated. The hole continues its path and will eventually be annihilated by the electron from figure 1. Because of the entanglement of the spins, the remaining electron takes on the state of the annihilated electron.

Teleportation by electron-hole annihilation

Back to the earthly electron. It falls in the hole and disappears in the Fermi sea. Its quantum mechanical state $\alpha\vert\!\uparrow\rangle+\beta\vert\!\downarrow\rangle$ was unknown and seems lost. The entanglement, however, acts like a "soul" that transfers the state from the earthly electron to the heavenly electron. Here is how it works: In order to fill the hole, the spin of the earthly electron and that of the hole have to line up. The entanglement ensures that this spin correlation is inherited by the heavenly electron. The spin of the heavenly electron is therefore no longer distributed isotropically, but has acquired the state $\alpha\vert\!\uparrow\rangle+\beta\vert\!\downarrow\rangle$ of the earthly electron. This instantaneous transfer of a quantum mechanical state between two distant particles is what Bennett et al. have called teleportation [1], with a nod to StarTrek.
Although teleportation is instantaneous, Einstein can rest assured: There is no instantaneous transfer of information. Since it is unpredictable when a tunneling attempt is successful, a message will need to be sent by regular (classical) means that the teleportation has happened. The distant electron can not measure whether its state is still isotropic or not, because any measurement will destroy the quantum mechanical state itself. The instantaneous transfer of the state from one to the other electron is necessary to satisfy the no-cloning theorem from quantum mechanics: At no instant does there exist more than a single copy of the state $\alpha\vert\!\uparrow\rangle+\beta\vert\!\downarrow\rangle$ .
Outlook

The state $\alpha\vert\!\uparrow\rangle+\beta\vert\!\downarrow\rangle$ is the qubit in a quantum computer. Teleportation makes it possible in principle to transport that state from one part of the electrical circuit to the other, without having to disturb that state by a measurement. That is the long-term motivation of our research. On the short term, it would be a major breakthrough if the entanglement of the electron-hole pair could be measured. Teleportation over a distance of a few micrometers would then be the logical next step. A small step, perhaps, for Captain Kirk, but a giant step for science.
A Dutch version of this article appeared in: Nederlands Tijdschrift voor Natuurkunde 70, 112 (2004).

[1] C.H. Bennett, G. Brassard, C. Crépeau, R. Jozsa, A. Peres, W.K. Wootters, Phys.Rev.Lett. 70, 1895 (1993).
[2] D. Bouwmeester, J.-W. Pan, K. Mattle, M. Eibl, H. Weinfurter, A. Zeilinger, Nature 390, 575 (1997).
[3] C.W.J. Beenakker, M. Kindermann, Phys.Rev.Lett. 92, 056801 (2004).
[4] C.W.J. Beenakker, C. Emary, M. Kindermann, J.L. van Velsen, Phys.Rev.Lett. 91, 147901 (2003).

Up: Topics